Moonshine

In the late 1970´s John McKay was working on the largest (sporadic) group which is called the monster group due to its large number of elements (more than 1053). The smallest three irreducible representations of the monster group are 1, 196 883 and 21 296 876 dimensional. When John McKay came across the J(q) function that enjoys the expansion

J(q) = 1/q + 196 884 q + 21 493 760 q2 + ...

he noticed that

196 884 = 196 883 + 1,
21 493 760 = 21 296 876 + 196 883 + 1.

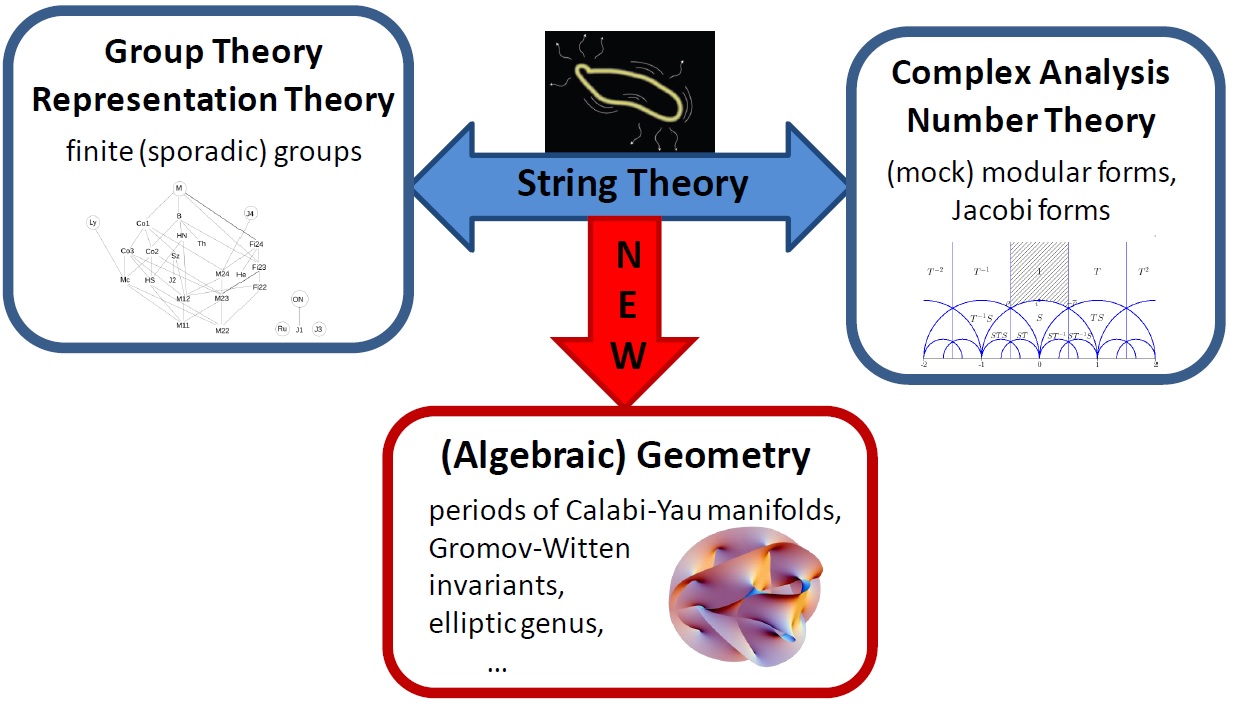
Such a pattern persists for all coefficient in the expansion of the J(q) and points towards a connection between the largest sporadic group, the monster, and the J(q) function. More general this connects the mathematical branches of Representation and Group Theory with Complex Analysis and Number Theory. This connection can be explained by string theory as follows:
A compactification of the bosonic string theory on a particular space has as its 1-loop partition function the J(q) function. This means that for this particular compactification of the bosonic string theory the trace over the (left-moving) states graded by their energy is

Z(q) = Tr ( qH) = J(q).

Additionally, one can show that the symmetry group of the internal space is the Monster group. Thus from this particular string compactification we can understand the connection between the Monster group and the J(q) function: The states of the string theory inherit the monster symmetry from the compact space and therefore we can decompose the coefficients of the partition function at any given energy level (i.e. any given power of q) into irreducible representations of the Monster group. The above connection is usually referred to as Monstrous moonshine.
There are a variety of further moonshine phenomea and recently it was possible, using string dualities, to establish a connection to a third branch of Mathematcs, namely (algebraic) geometry: